Determinism in Quantum Slit-Experiments
Quantum
DOI:
https://doi.org/10.55672/hij2022pp115-121Keywords:
Quantum mechanics, System theory, Determinism, Causality, ModellingAbstract
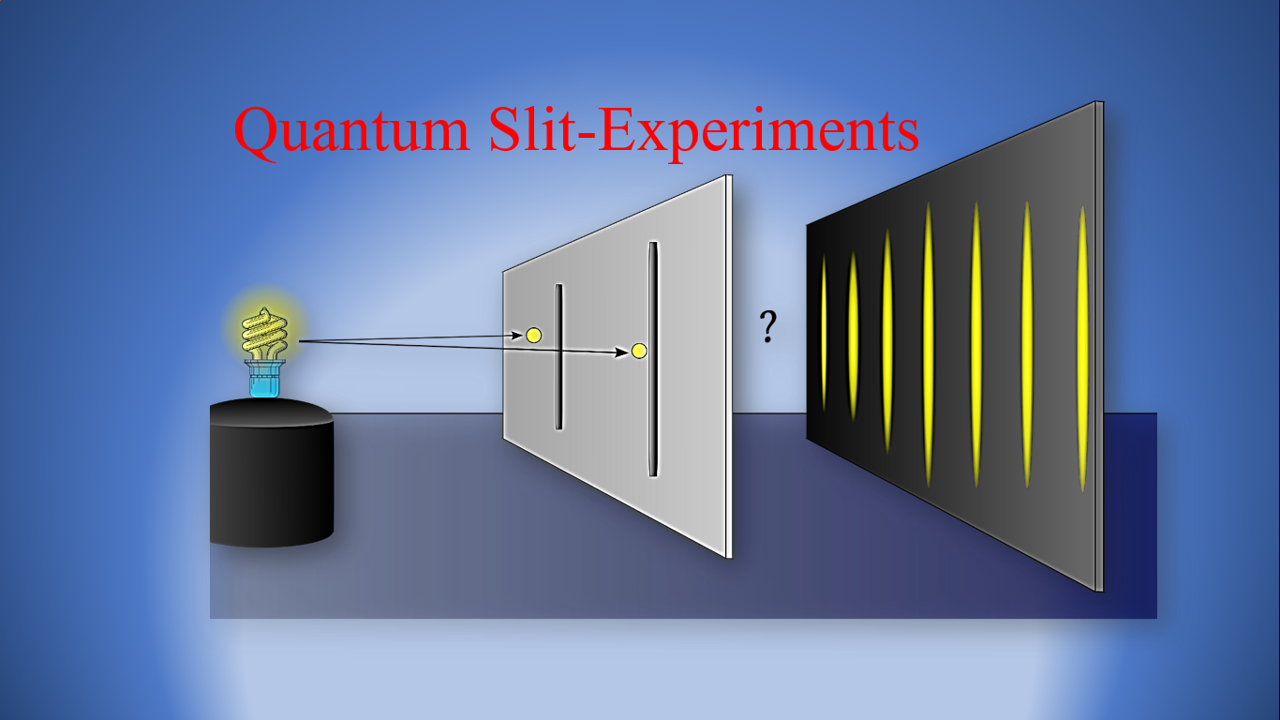
A mathematical model for the slit experiments in the heart of quantum mechanics is developed to gain insight into quantum theory. The proposed system-theoretical model is entirely based on commutative mathematics, i.e. convolution, and integral transformations, and starts with spacetime functions with inherent energy-based cause and effect relations of the state-function Ѱ in the complex Hilbert space. The benefits of his approach are as 1-Invariance in time reversal. 2-Deterministic result functions in the model in line with the outcome of slit experiments. 3- Separation of causality and cross-correlations of attained states. 4- Disappearance of a posteriori probability of quantum states. 5- Quantum a priori fixed states after causality interactions have ended, (even) when quanta are (light-years) separated. The model predicts the patterns in the experiments with mathematical functions of the energy distributions. The quantum mechanical counterpart description of the physical reality of slit experiments thus may be considered complete in A. Einstein’s definition. The patterns in double slit experiments are found to be an effect of energy (amplitude-) modulation. An equivalent double-slit pattern can be retrieved from an input modulated 1-slit experiment excluding interference interpretations. The system-theoretical model uses generic properties of quanta and evolves into determinism in quantum mechanics slit experiments. The mathematics in the model handles beables by treatment of momentum p in system theoretical I/O relations of the transformed functions and allows the proposed description by the avoidance of a direct addressing of the individual quanta through variables. The following method yields exact, non-probabilistic results.
Downloads
Author Biography
Albert. V. Herrebrugh, Netherland According to the request of the author of this article, the affiliation has been secured with Hyperscience International Journal
Albert. V. Herrebrugh 
Netherland
According to the request of the author of this article, the affiliation has been secured with Hyperscience International Journal
References
[1] R. P. Feynman, R. B. Leighton, and M. Sands, The Feynman lectures on physics, Vol. I: The new millennium edition: mainly mechanics, radiation, and heat. Basic books, 2011.
[2] L. J. N. l. De Broglie, "The wave nature of the electron," vol. 12, pp. 244-256, 1929.
[3] I. H. Blok, "Integraal transformaties in de elektrotechniek," in collegeleidraad, ed, 1973.
[4] J. S. Bell, "The theory of local beables," 1975.
[5] A. Einstein, B. Podolsky, and N. J. P. r. Rosen, "Can quantum-mechanical description of physical reality be considered complete?," vol. 47, no. 10, p. 777, 1935.
[6] M. J. Lighthill, M. S. Lighthill, and M. J. Lighthill, An introduction to Fourier analysis and generalised functions. Cambridge University Press, 1958.
[7] j. Macki, "bremermann, h-distributions complex variables and fourier transforms," ed: canadian mathematical soc 577 king edward rd, po box 450, station a, ottawa …, 1966.
[8] R. A. Roberts, Signals and linear systems. Wiley, 1973.
[9] J. G. Truxal, Introductory system engineering. McGraw-Hill College, 1972.
[10] W. Heisenberg, "The Physical Principles of the Quantum Theory (tr. Eckhart, K., Hoyt, FC)," ed: Dover, New York, NY, USA (rpt. 1949), 1930.
[11] P. Dirac, "The principles of quantum mechanics, 4th (rev.) edn," ed: Oxford: Clarendon Press, 1930.
[12] G. J. F. i. P. 't Hooft, "Time, the arrow of time, and quantum mechanics," vol. 6, p. 81, 2018.
Published
How to Cite
Issue
Section
License
Copyright (c) 2022 Hyperscience International Journal
This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
